End-of-chapter problems. Solutions to problem Set a

Solutions to Problem Set A

9-1A. (a) IO = FCFt[PVIFIRR%,t yrs]

$10,000 = $17,182 [PVIFIRR%,8 yrs]

0.582 = PVIFIRR%,8 yrs

Thus, IRR = 7%

(b) $10,000 = $48,077 [PVIFIRR%,10 yrs]

0.208 = PVIFIRR%,10 yrs

Thus, IRR = 17%

(c) $10,000 = $114,943 [PVIFIRR%,20 yrs]

0.087 = PVIFIRR%,20 yrs

Thus, IRR = 13%

(d) $10,000 = $13,680 [PVIFIRR%,3 yrs]

.731 = PVIFIRR%,3 yrs

Thus, IRR = 11%

9-2A. (a) I0 = FCFt[PVIFAIRR%,t yrs]

$10,000 = $1,993 [PVIFAIRR%,10 yrs]

5.018 = PVIFAIRR%,10 yrs

Thus, IRR = 15%

(b) $10,000 = $2,054 [PVIFAIRR%,20 yrs]

4.869 = PVIFAIRR%,20 yrs

Thus, IRR = 20%

(c) $10,000 = $1,193 [PVIFAIRR%,12 yrs]

8.382 = PVIFAIRR%,12 yrs

Thus, IRR = 6%

(d) $10,000 = $2,843 [PVIFAIRR%,5 yrs]

3.517 = PVIFAIRR%,5 yrs

Thus, IRR = 13%

9-3A. (a) $10,000 = + +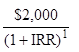

Try 18%:

$10,000 = $2,000(0.847) + $5,000 (0.718) + $8,000 (0.609)

= $1,694 + $3,590 + $4,872

= $10,156

Try 19%

$10,000 = $2,000 (0.840) + $5,000 (0.706) + $8,000 (0.593)

= $1,680 + $3,530 + $4,744

= $9,954

Thus, IRR = approximately 19%

(b) $10,000 = + +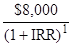

Try 30%

$10,000 = $8,000 (0.769) + $5,000 (0.592) + $2,000 (0.455)

= $6,152 + $2,960 + $910

= $10,022

Try 31%:

$10,000 = $8,000 (0.763) + $5,000 (0.583) + $2,000 (0.445)

= $6,104 + $2,915 + $890

= $9,909

Thus, IRR = approximately 30%

(c) $10,000 = +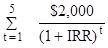

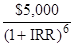
Try 11%

$10,000 = $2,000 (3.696) + $5,000 (0.535)

= $7,392 + $2,675

= $10,067

Try 12%

$10,000 = $2,000 (3.605) + $5,000 (0.507)

= $7,210 + $2,535

= $9,745

Thus, IRR = approximately 11%

9-4A. (a) NPV = 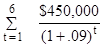- $1,950,000

= $450,000 (4.486) - $1,950,000

= $2,018,700 - $1,950,000 = $68,700

(b) PI =

= 1.0352

(c) $1,950,000 = $450,000 [PVIFAIRR%,6 yrs]

4.333 = PVIFAIRR%,6 yrs

IRR = about 10% (10.1725%)

(d) Yes, the project should be accepted.

9-5A. (a) Payback Period = $80,000/$20,000 = 4 years

Discounted Payback Period Calculations:

Cumulative

Undiscounted Discounted Discounted

Year Cash Flows PVIF10%,n Cash Flows Cash Flows

	-$80,000	1.000	-$80,000	-$80,000
	20,000	.909	18,180	-61,820
	20,000	.826	16,520	-45,300
	20,000	.751	15,020	-30,280
	20,000	.683	13,660	-16,620
	20,000	.621	12,420	-4,200
	20,000	.564	11,280	7,080

Discounted Payback Period = 5.0 + 4,200/11,280 = 5.37 years.

(b) NPV = 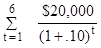- $80,000

= $20,000 (4.355) - $80,000

= $87,100 - $80,000 = $7,100

(c) PI =

= 1.0888

(d) $80,000 = $20,000 [PVIFAIRR%,6 yrs]

4.000 = PVIFAIRR%,6 yrs

IRR = about 13% (12.978%)

9-6A. (a) NPVA = 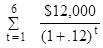- $50,000

= $12,000 (4.111) - $50,000

= $49,332 - $50,000 = -$668

NPVB = 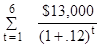- $70,000

= $13,000 (4.111) - $70,000

= $53,443 - $70,000 = -$16,557

(b) PIA =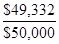

= 0.9866

PIB =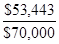

= 0.7635

(c) $50,000 = $12,000 [PVIFAIRR%,6 yrs]

4.1667 = PVIFAIRR%,6 yrs

IRRA = 11.53%

$70,000 = $13,000 [PVIFAIRR%,6 yrs]

5.3846 = PVIFAIRR%,6 yrs

IRRB = 3.18%

Neither project should be accepted.

9-7A. (a) Project A:

Payback Period = 2 years + $100/$200 = 2.5 years

Project A:

Discounted Payback Period Calculations:

Cumulative

Undiscounted Discounted Discounted

Year Cash Flows PVIF10%,n Cash Flows Cash Flows

	-$1,000	1.000	-$1,000	-$1,000
		.909		-455
		.826		-207
		.751		-57
		.683
		.621

Discounted Payback Period = 3.0 + 57/68 = 3.84 years.

Project B:

Payback Period = 2 years + $2,000/$3,000 = 2.67 years

Project B:

Discounted Payback Period Calculations:

Cumulative

Undiscounted Discounted Discounted

Year Cash Flows PVIF10%,n Cash Flows Cash Flows

	-$10,000	1.000	-$10,000	-$10,000
	5,000	.909	4,545	-5,455
	3,000	.826	2,478	-2,977
	3,000	.751	2,253	-724
	3,000	.683	2,049	1,325
	3,000	.621	1,863	3,188

Discounted Payback Period = 3.0 + 724/2,049 = 3.35 years.

Project C:

Payback Period = 3 years + $1,000/$2,000 = 3.5 years

Project C:

Discounted Payback Period Calculations:

Cumulative

Undiscounted Discounted Discounted

Year Cash Flows PVIF10%,n Cash Flows Cash Flows

	-$5,000	1.000	-$5,000	-$5,000
	1,000	.909		-4,091
	1,000	.826		-3,265
	2,000	.751	1,502	-1,763
	2,000	.683	1,366	-397
	2,000	.621	1,242

Discounted Payback Period = 4.0 + 397/1,242 = 4.32 years.

Project	Traditional Payback	Discounted Payback
A	Accept	Reject
B	Accept	Reject
C	Reject	Reject

9-8A. NPV9% = 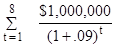- $5,000,000

= $1,000,000 (5.535) - $5,000,000

= $5,535,000 - $5,000,000 = $535,000

NPV11% = 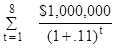- $5,000,000

= $1,000,000 (5.146) - $5,000,000

= $5,146,000 - $5,000,000 = $146,000

NPV13% = 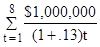- $5,000,000

= $1,000,000 (4.799) - $5,000,000

= $4,799,000 - $5,000,000 = -$201,000

NPV15% = 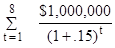- $5,000,000

= $1,000,000 (4.487) - $5,000,000

= $4,487,000 - $5,000,000 = -$513,000

9-9A. Project A:

$50,000 = + +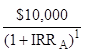

+ +

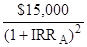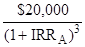
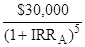
Try 23%

$50,000 = $10,000(.813) + $15,000(.661) + $20,000(.537)

+ $25,000(.437) + $30,000(.355)

= $8,130 + $9,915 + $10,740 + $10,925 + $10,650

= $50,360

Try 24%

$50,000 = $10,000(.806) + $15,000(.650) +$20,000(.524)

+ $25,000(.423) + $30,000(.341)

= $8,060 + $9,750 + $10,480 + $10,575 + $10,230

= $49,095

Thus, IRR = just over 23%

Project B:

$100,000 = $25,000 [PVIFAIRR%,5 yrs]

4.00 = PVIFAIRR%,5 yrs

Thus, IRR = 8%

Project C:

$450,000 = $200,000 [PVIFAIRR%,3 yrs]

2.25 = PVIFAIRR%,3 yrs

Thus, IRR = 16%

9-10A. (a) NPV = 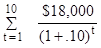- $100,000

= $18,000(6.145) - $100,000

= $110,610 - $100,000

= $10,610

(b) NPV = 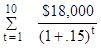- $100,000

= $18,000(5.019) - $100,000

= $90,342 - $100,000

= -$9,658

(c) If the required rate of return is 10% the project is acceptable as in part (a).

(d) $100,000 = $18,000 [PVIFAIRR%,10 yrs]

5.5556 = PVIFAIRR%,10 yrs

IRR = Between 12% and 13% (12.41%)

9-11A. (a) =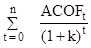

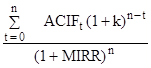
$10,000,000 =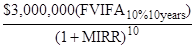

$10,000,000 =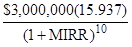

$10,000,000 =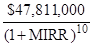

MIRR = 16.9375%

(b) $10,000,000 =

$10,000,000 =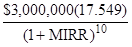

$10,000,000 =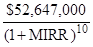

MIRR = 18.0694%

(c) $10,000,000 =

$10,000,000 =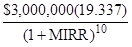

$10,000,000 =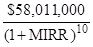

MIRR = 19.2207%

Дата добавления: 2015-10-30