L – is a difference between h and L

Ph – is a size of the painting

θ – viewers angle on the painting

β – viewers angle on the distance between painting and eye level

α – θ + β, angle from eye level to the highest point of the painting

As it is clearly provided on the figure 29 a triangle with α angle has a straight angle, triangle with β angle has a straight angle as well, the wanted angle which should be maximised is θ, but this angle is a part of the triangle which does not have a straight angle. A straights angle is to find a formula for unknown angle through triangles sides – opposite, adjacent and hypotenuse.

Sin = Cos = Tan =

So to find α we will need two sides: size of the painting + is a difference between h and L – opposite side and distance between person and painting – adjacent side which is x and should be found as well. Formula for the α will be:

α = Tan^-1 (

α = Tan^-1 (

Knowing that α is a sum of two smaller triangles we can find θ triangle trough this pattern. As triangle with β angle has a straight angle as well as α triangle We can work through Tan formula to find β

β = Tan^-1 (

β = Tan^-1 (

Now it is possible to find θ through α taking β

θ = α – β

θ = Tan^-1 ( Tan^-1 (

If to denote θ as f(x) there will be an equation which will help to find maximised x to make a maximised θ

f(x) = Tan^-1 ( Tan^-1 (

To use an application to optimisation problems and finding maxima of the curve to state a maximised distance for finding maximised angle the investigated equation will need to be differentiated

Differentiate formula for Tan^-1(x):

Let y= Tan^-1(x)

Where

Then let Tan y = x

Using implicit differentiation and solving for dv/dx

When 1+Tan² y = sec² y

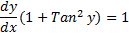
When x = Tan y

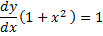
So

Differentiated f(x) = Tan^-1 ( Tan^-1 ( formula will be

f ‘(x) =

Investigated formula will help to find maximised angle for all cases when h is less then L

Figure 30

I^st Scenario

x - is a distance between person and painting

h – is a height of the eye level of the person – 150 cm and 200 cm

L – as a height placement of the painting on the wall – 160 cm

l – is a difference between h and L – 10 cm or 40 cm more

Ph – is a size of the painting - 80

θ – viewer’s angle on the painting

β – viewer’s angle on the distance between painting and eye level

α – θ + β, angle from eye level to the highest point of the painting

Figure 30 represents a case when person’s height is 150 cm

Figure 31

By substituting values to the equation we will find a maximised distance for the person with 150 cm height

f(x) = Tan^-1 ( Tan^-1 (

f(x) =Tan^-1 (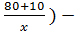 Tan^-1 (

f(x) = Tan^-1 ( Tan^-1 (

Figure 31 clearly shows that Maximum point is between x=25 and x=35

f ‘(x) =

f ‘(x) =

Figure 32

when f ‘(x)=0

x= -30 and x=30 (figure 32)

We need only positive number as we need maximised value

So when distance from the picture is 30 cm person will see the picture with maximised angle, we will find this angle through already found x value.

θ = Tan^-1 ( Tan^-1 (

θ = 0.9272 Radians

θ = 0.9272 = 51.5662ﾟ

Figure 33

For the case when person is 200 cm high the angle and distance should be different because person’s height is bigger than a height placement of the painting on the wall. (figure 33)

In this case α is in front of the size of the painting – 80 cm

But other and very important point is that θ triangle has a straight angle but it is now not a full angle of the view

Figure 34

Height of the person is 200 cm, height placement is 160 cm, so person looks straight to the centre of the picture and this means that opposite side of the θ triangle is 40 cm

α = 2Tan^-1 (

Formula for finding θ will be: (Figure 34)

θ = Tan^-1 (

Differentiated formula will be

θ’ =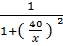

β is a straight triangle as well and its opposite side is 40 cm

it is reasonable to state that α will be 2 θ

α = 2 Tan^-1 (

α’=

α’ =

Figure 35

at α’ = 0 equation has an error

x	α’ =
-1	0.000083
	undefined
	0.000083

This means that the angle will decrease by moving from the painting and the optimised viewing angle is 180ﾟbut this is not possible in real life, so

At x=1 θ will be:

α = 2 Tan^-1 (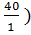

α = 177 ﾟ

At the case when person’s eye level height is 150 optimised distance from the painting is 30 cm and angle is 51.5ﾟ; for the case when person’s eye level is at some point at the painting maximised angle should be 180ﾟ, but it is not impossible so the least distance for the viewer can be 1 cm, at one cm the viewing and is 177ﾟ.

Figure 36

At the painting’s size 80 cm and 160 cm of height placement optimised distance for people with height from 150 cm to 200 cm is from 1 cm to 30 cm, the angle will be from 51.5ﾟ to 177ﾟ

Дата добавления: 2015-10-29