A. functions with combination of features

Figure 26

To get few combinations in one curve I will summarize two functions: with a cusp and a function with one point of inflection, one maxima and one minima

f(x)=

f(x)=

To get proper curve proper values should be used. To make a curve with one point of inflection, one maxima and one minima with a shift along the x-axis, both and fractions need to have the same shift, otherwise the curve of the subtraction of the functions will not have a proper shape (Figure 26)

Figure 27

f(x)=

f(x)=

and f(x)= functions have different stationary point values, they do not match so the curve of their sum has no point of inflection when f(x)= values take f(x)= values

f(x)=

The graph I want will be:

f(x)=

f(x)=

f(x)=

a curve with one point of inflection, one maxima and one minima with a shift to the right by two values along the x-axis (Figure 27)

To add a cusp curve to the

f(x)= function the cusp curve should have the same shift along the x-axis because the cusp and the point of inflection should coincide with each other or cusp needs to coincide with any point of the other curve to create a cusp in a new curve

All functions can be modified in different cases, but in the situation when I want to get a cusp, and at least some other clear points I will create a cusp in the middle and use a normal cusp curve f(x)= to clearly outline newly created stationary points.

Figure 28

Two functions: f(x)= and f(x)= will be combined by subtraction

f(x)=

f(x)=

f(x)=

+1 at the end of the equation shifts all curves up by one value (Figure 28)

f(x)=

Newly created graph has four stationary points:

f(x)=

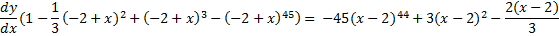
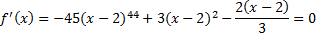
X= 2

X=1.0577

X= 2.2222

X= 2.93149

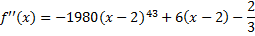
- (2) = - 2/3 (local max)

- (1.0577)= 147.4243 (local min)

- (2.2222)= 0.6665 (local min)

- (2.93149)= -88.68889 (local max)

At x=2 the curve has a cusp, and at x=1.0577 (local min), x=2.2222 (local min), x=2.93149 (local max)

(Complex calculations were made at http://www.wolframalpha.com/)

Question 5

Gumnut gallery

This report will investigate the distance viewers should be advised to stand away from a wall on which paintings are hung in order to optimise their viewing angle

Figure 29

To find the maximised angle we need do investigate a formula which will work for all cases and scenarios

Figure 29 has a sketch of few patterns:

x - is a distance between person and painting

h – is a height of the eye level of the person

L – as a height placement of the painting on the wall

Дата добавления: 2015-10-29