These three cases are next treated in more detail.

(a) Consider the dislocations randomly oriented with an average sep­aration of 2Z. If \/:;l_it > 6Z then a diffusing atom encounters several dislocations over the time t, and the effect of the dislocations is to increase the average jump frequency of the atoms in an isotropic man­ner, thus D_eff/D_i > 1. If the heat flow analogy of the grain boundary diffusion problem was a system consisting of a sheet of aluminum foil between two sheets of plastic, then the heat flow analogy here would be a system consisting of fine aluminum wires randomly distributed in plastic. The relation between Deff and D_I can be obtained by a random walk argument due to Hart." Assume that each atom makes n jumps in a pure metal single crystal containing many randomly oriented dis­locations. Ignoring correlation effects and assuming all jumps are of length r, the net displacement for each atom after n jumps is R, and the average displacement

204 Diffusion in Solids

R², = nr² (6-12) Now of the n jumps taken by an atom n₁ were in the lattice and n_p in dislocation pipes. The jump frequencies inside and outside the dislo­cation are defined as

t_P F = n _p and t_i = ni

where n =- n_p + n₁, t_p and t₁ are the times spent in the dislocation and
lattice respectively, and t = t_p + t₁. Eq. (6-12) can then be rewritten
Rt_i

(6-13)

t

P = to _r. _
^{I r} Except for a geometric constant, R_n²/t equals _Deff; and except for the

same constant, Fp r² and T_I r² equal D_p and D₁, respectively. Thus Deff for a single crystal is

Deff gD_p + D₁(1-g)' D₁(1 + g(D_p/D₁)) (6-14)

where g = t_p/t. For a pure metal g is (a/Z)², or the fraction of atoms in the pipe. Some straightforward arithmetic indicates that if D_p/D, = 10⁷ then the dislocations in an annealed crystal (10⁶/cm²) have a negligible effect on D_er, but if the dislocation density is increased a hundred fold by slight cold working, Deff is increased significantly. For the diffusion of a solute that tends segregate to the dislocation, g = K(a/Z)².

(b) In this case there is a region well below the surface in which the lattice around each dislocation pipe contains solute which entered by diffusion along the dislocation, that is VD7t a. However, these solute fields do not overlap with one another because, //7₁t < Z. Thus a semilogarithmic plot of the average concentration e(y) in a section at a depth y beneath the surface will show two regions. Near the sur­face where lattice diffusion is dominant ln(C) is proportional to y² and the line has a slope (-1/4D₁t). Beneath this at a depth of say y > 5\/D ₁t, ln(C) is proportional to y (not y^6/5 as in the case of grain boundary diffusion) and the slope is given by

d ln(C)Idy = Ana(D_p/D₁)^1/21 with A ~ 0.8 (6-15)

This slope is independent of time, in distinct contrast to the case for a tail due to grain boundary diffusion (see Eq. 6-6). Also, the slope is independent of: the density of dislocations, and whether the surface concentration is held constant or a thin film is applied. However, the intercept of a plot of In (c) vs. y extrapolated to y =-- 0 would depend on each of these variables.

200 300

Y (pm)

Fig. 6-12—Penetration curve for Na* into an NaC1 single crystal showing a tail due to diffusion along dislocations. (Y. K. Ho, Thesis, Imperial College, London, 1982. See LeClaire & Rabinovitch)

Fig. 6-12 shows two penetration plots for a Na tracer diffusing from a thin surface film into pure NaC1 single crystals in the low temperature (extrinsic) range. Note that the linear plot fits the data quite well, and that increasing the time by a factor of 4 does not change the slope. Both of these observations confirm that the tail is due to separate dis­locations not dislocations aligned in low angle boundaries. Ho also treated the contribution of these dislocations to the electrical conduc­tivity and found that

= (F²D/RTf)Tr[a²(D_p/D_i)ld (6-16)

where d is the dislocation density. This adds one more mechanism to the list of possibilities that can influence o^- and D in the Extrinsic range.

(c) Here the solute all stays in the dislocation pipes, and e_r(y,t) is obtained in the same way used in Chap. 1 with D_p replacing D.

6.4 DIFFUSION ALONG MOVING BOUNDARIES

Our treatment of grain boundary diffusion has been limited to sta­tionary grain boundaries. There the transport from the surface into the lattice is aided by boundary diffusion, but ultimately limited by dif­fusion through the lattice. However, if the boundary moves as diffu­sion occurs, the rate of mixing from the surface into the lattice is greatly increased. Recently it has become clear that in samples containing strong concentration gradients diffusion along existing grain boundaries can induce the boundaries to move, and even induce nucleation and growth of new grains. The difference in the degree of mixing of solute in the two cases can be profound. The difference in solute distribution be­tween stationary and moving boundaries is indicated in Fig. 6-13. There at the boundary that has not moved the solute enriched layer is deep and very thin. The solute enrichment behind the boundary that has moved is somewhat more shallow, but orders of magnitude wider.

The sort of behavior shown in Fig. 6-13 is not found when the con­centration difference between the surface layer and bulk is small, but is quite common if the concentration is large. It is called, Diffusion

Fig. 6-13—Cross section of sample containing two grain boundaries after exposure of the upper surface to a solute vapor. One boundary has exhibited DIGM along a portion of its length, one has not. The shaded area reflects the relative volume of solute en­riched matrix around the two boundaries.

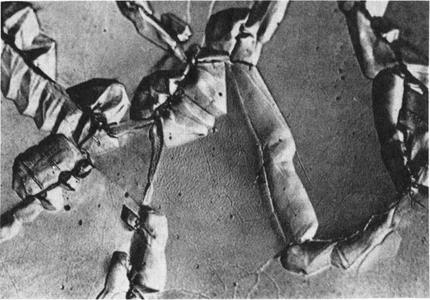
Fig. 6-14—Photomicrograph illustrating the surface relief accompanying the local zincification of an iron foil 40 microns thick by DIGM. [M. Hillert, G. Purdy, Acta Met. 26 (1978) 333.]

Induced Grain-boundary Migration (DIGM). One of the systems which easily exhibits DIGM is Fe-Zn. Fig. 6-14 shows the surface of a sam­ple which has undergone DIGM. The regions high in Zn show up along the original boundaries in relief and contained 6-7% Zn through the entire thickness of the foil. The remaining part of the grains showed no increase in Zn aside from a surface layer about 0.1 urn deep formed by lattice diffusion. The solute rich regions shown in Fig. 6-14 formed both by the movement of existing boundaries, and the nucleation and growth of new grains.

Questions concerning DIGM can be grouped in three categories: what happens, why, and when? The first has been touched on above, and will be described further below. As for `why?', DIGM is driven by the free energy of mixing solute into the lattice. There is no general agreement on how this free energy couples to the boundary to move it, and indeed there may be several mechanisms operating at different times and in different systems.¹⁵ The central point for our purpose is that DIGM can occur in alloy systems, and when it does, it will greatly increase the apparent D.

The question of when DIGM occurs has several types of answers:

• DIGM has been observed in essentially all binary alloys where it has been looked for. It has been reported in a few binary ceramic sys­tems, but has not been found in most ceramic systems investigated.

· DIGM has been reported to occur over a range of temperatures up to almost the melting point, but the effect is most pronounced and important at low temperatures.

· Only high angle boundaries exhibit DIGM. This may be due to their higher values of Db, but probably has more to do with their ability to generate the many new sites needed to incorporate the solute into the alloy lattice.

· The movement is not continuous in time for a given boundary, but may stop and start again, may stop and then reverse, or may stop and not start again.

· The rate of both nucleation and growth increases as the Ac across the boundary increases.

---

Дата добавления: 2015-10-29