Dilution 1:10 dilution 1:10 dilution

Specimen Preparation and Processing

The most frequently encountered specimen in immunological testing is serum. Blood is collected aseptically by venipuncture into a clean, dry, sterile tube. Care must be taken to avoid hemolysis, since this may produce a falsepositive test. The blood specimen is allowed to clot at room temperature or at 4°C and then centrifuged. Serum should be promptly separated into another tube without transferring any cellular elements. Fresh, nonheat inactivated serum is usually recommended for testing. However, if testing cannot be performed immediately, serum may be stored between 2°C and 8°C for up to 72 hours. If there is any additional delay in testing, the serum should be frozen at –20°C or below.

Simple Dilutions

For many tests, a measured amount of a serum sample is used directly for detection of antibodies. However, in order for a visible end point to occur in a serological reaction, the relative proportions of antigen and antibody present are important. Sometimes in a serological test, too much antibody may be present, and an end point may not be reached. In this case, serum that contains antibody must be diluted. Therefore, knowledge of serial dilutions is essential to understanding all serological testing in the laboratory.

A dilution involves two entities: the solute, which is the material being diluted, and the diluent, which is the medium making up the rest of the solution. The relationship between these two is expressed as a fraction. For example, a 1:20 dilution implies 1 part of solute and 19 parts of diluent. The number on the bottom of the fraction is the total volume, reached by adding the volumes of the solute and diluents together.

1/Dilution = Amount of Solute/Total Volume

To create a certain volume of a specified dilution, it is helpful to know how to manipulate this relationship. An algebraic equation can be set up to find the total volume, the amount of solute, or the amount of diluent needed to make a dilution. Consider the following example:

ML of a 1:20 dilution is needed to run a specific serological test. How much serum and how much diluent are needed to make this dilution?

The equation is set up using the fraction for the dilution, indicating the relationship between the total volume and the solute, or the amount of serum needed:

1/20 = x /2 mL

Note that the 20 represents the total number of parts in the solution and that 2 mL is the total volume desired.

Solving this equation for x gives 0.1 mL for the amount of serum needed to make this dilution. The amount of diluents is obtained by subtracting 0.1 mL from 2.0 mL to give 1.9 mL of diluent. To check the answer, simply set up a proportion between the amount of solute over the total volume. This should equal the dilution desired. Thus, the correct answer has been obtained.

If, on the other hand, one knows the amount of serum to be used, a problem can be set up in the following manner:

A 1:5 dilution of patient serum is necessary to run a serological test. There is 0.1 mL of serum that can be used. What amount of diluent is necessary to make this dilution using all of the serum?

A slightly different formula can be used to solve this problem:

1/Dilution – 1 = Amount of Solute/Amount of Diluent

1⁄4 = 0.1 mL/ x

x = 0.4 mL of diluent

Note that the final volume is obtained by adding 0.1 mL of solute to the 0.4 mL of diluent. Dividing the volume of the solute by the total volume of 0.5 mL yields the desired 1:5 ratio.

Depending on the unknown being solved for, either of these formulas can be used. To calculate the total volume, the total dilution factor must be used. If, however, the amount of diluent is to be calculated, the formula using dilution 21 can be used. Further problems are given at the end of the chapter to allow practice with dilution calculations.

Compound Dilutions

The previous examples represent simple dilutions. Occasionally in the laboratory it is necessary to make a very large dilution, and it is more accurate and less costly to do this in several steps rather than all at once. Such a process is known as a compound dilution. The same approach is used, but the dilution occurs in several stages. For example, if a 1:500 dilution is necessary, it would take 49.9 mL of diluents to accomplish this in one step with 0.1 mL of serum. If only a small amount of solution is needed to run the test, this is wasteful; furthermore, inaccuracy may occur if the solution is not properly mixed. Therefore, it is helpful to make several smaller dilutions.

To calculate a compound dilution problem, the first step is to plan the number and sizes of simple dilutions necessary to reach the desired end point. To use the preceding example, a 1:500 dilution can be achieved by making a 1:5 dilution of the original serum, a 1:10 dilution from the first dilution, and another 1:10 dilution. This can be shown as follows:

Serum:

dilution 1:10 dilution 1:10 dilution

0.1 mL serum 0.1 mL of 1:5 dilution 0.1 mL of 1:10 dilution

0.4 mL diluent 0.9 mL diluent 0.9 mL diluents

Multiplying 5 x 10 x 10 equals 500, or the total dilution. Each of the simple dilutions is calculated individually by doing mental arithmetic or by using the formula given for simple dilutions. In this example, the 1:500 dilution was made using very little diluent in a series of test tubes, rather than having to use a larger volume in a flask. The volumes were kept small enough so that mixing could take place easily, and the final volume of 1.0 mL is all that is necessary to perform a test.

If, in each step of the dilution, the dilution factor is exactly the same, this is known as a serial dilution. Serial dilutions are often used to obtain a titer, or indicator of an antibody’s strength. A series of test tubes is set up with exactly the same amount of diluent in each (Fig. 1). The most common serial dilution is a doubling dilution, in which the amount of serum is cut in half with each dilution. For example, six test tubes can be set up with 0.2 mL of diluents in each. If 0.2 mL of serum is added to the first tube, this becomes a 1:2 dilution.

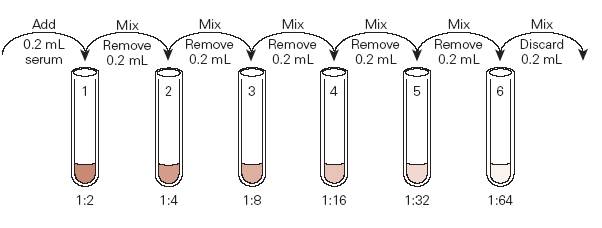
FIGURE 1. Serial dilution. Each tube contains 0.2 mL of diluent. Patient serum (0.2 mL) is added to tube one. This is carefully mixed, and then 0.2 mL is withdrawn and added to tube two. The process is continued until the last tube is reached. The sample is mixed, and 0.2 mL is discarded. Note that in this dilution, the amount of antibody is cut in half in each successive tube.

0.2 mL serum/0.2 mL serum + 0.2 mL

diluent = 0.2 mL/0.4 mL = ½

Then when 0.2 mL of the 1:2 dilution is added to 0.2 mL of diluent, a 1:4 dilution is obtained. The final dilution is obtained by counting the number of tubes and setting up a multiplication series in which the original dilution factor is raised to a power equal to the number of tubes. In this example, if the first tube contains a 1:2 dilution, the dilution in tube number six is

1⁄2 x 1⁄2 x 1⁄2 x 1⁄2 x 1⁄2 x 1⁄2 = 1/64

If, in this instance, an end point was reached at tube number five, the actual titer would be 1:32. To avoid confusion, this is customarily written as the reciprocal of the dilution – that is, 32. Serial dilutions do not always have to be doubling dilutions. Consider the following set of test tube dilutions:

1:5→1:25→1:125→1:625→1:3125

For each successive tube, the dilution is increased by a factor of 5, so this would indeed be considered a serial dilution. Having the ability to work with simple and compound dilutions and interpret serial dilutions is a necessary skill for laboratory work.

Principle

Serial dilutions are a set of dilutions in which the dilution factor is exactly the same at each step. These are used to make high dilutions with a small number of test tubes and a minimal amount of diluent. This is commonly done to determine the strength or titer of a particular antibody in patient serum as a part of the diagnosis of a disease state. Traditionally, serological pipettes have been used in this process, but now it is more common to employ micropipettes for this purpose. In this experiment, a series of doubling dilutions will be made with both serological pipettes and micropipettes, and the results will be compared.

Дата добавления: 2015-10-26