
Читайте также:
|
L E C T U R E 6
An operator  mapping a plane (or just a mapping of plane)
mapping a plane (or just a mapping of plane)  on a plane
on a plane 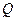 is a rule by which every point of plane
is a rule by which every point of plane  is put a unique point of the plane
is put a unique point of the plane 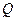 . A mapping is denoted as follows:
. A mapping is denoted as follows:  . If a point
. If a point  of a plane
of a plane  is transferred to a point
is transferred to a point 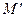 of a plane
of a plane 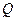 , we denote this by
, we denote this by 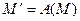 , and
, and 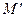 is the image of
is the image of  ,
,  is a pre-image of
is a pre-image of 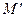 .
.
A mapping  is a bijection ( or bijective) if every point of the plane
is a bijection ( or bijective) if every point of the plane 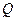 has a unique pre-image. A mapping that is inverse to a bijective mapping
has a unique pre-image. A mapping that is inverse to a bijective mapping  is the mapping
is the mapping 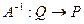 such that for every point
such that for every point  of the plane
of the plane  the following holds:
the following holds: 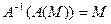 . A mapping
. A mapping  of plane
of plane  to itself is called a transformation of the plane
to itself is called a transformation of the plane  . A consecutive realization of transformations
. A consecutive realization of transformations 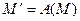 and
and 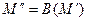 is called the product (or composition) of these transformations. The product of operators is written as
is called the product (or composition) of these transformations. The product of operators is written as 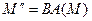 .
.
A point of a plane  transferred by a transformation
transferred by a transformation  to itself is called a fixed (immovable) point for
to itself is called a fixed (immovable) point for  . A set of the plane
. A set of the plane  consisting of fixed points for
consisting of fixed points for  is called fixed for
is called fixed for  . A set of points of
. A set of points of  transferred by
transferred by  to itself is called an invariant set of the transformation
to itself is called an invariant set of the transformation  .
.
Let in a plane with Cartesian system of coordinates 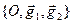 every its point
every its point  be put a unique point
be put a unique point 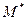 , i.e. a transformation of the plane be given. Let radius-vectors of these points be
, i.e. a transformation of the plane be given. Let radius-vectors of these points be 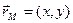 and
and 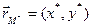 . Then the coordinates
. Then the coordinates 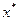 and
and 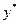 are some functions of
are some functions of  and
and 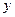 .
.
An operator 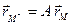 is linear if in every Cartesian system of coordinates
is linear if in every Cartesian system of coordinates 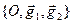 it is given by the formulas:
it is given by the formulas:  .
.
A linear operator can be written in matrix form:  where
where 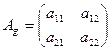 is the matrix of the linear operator
is the matrix of the linear operator  in the Cartesian system of coordinates
in the Cartesian system of coordinates 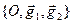 .
.
A linear operator  mapping a plane to itself with matrix
mapping a plane to itself with matrix 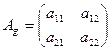 for which in every basis
for which in every basis  is called an affine transformation of the plane.
is called an affine transformation of the plane.
Theorem 1. If a linear transformation has regular matrix in some Cartesian system of coordinates then the matrix of this transformation will be regular in any other Cartesian systems of coordinates.
Theorem 2. Every affine transformation has the inverse transformation that is unique.
Proof. Since  , the system of linear equations
, the system of linear equations 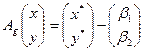 has a unique solution
has a unique solution  for every vector
for every vector  . It means that for every image of an affine transformation there is a unique pre-image, i.e. there is the inverse transformation. ÿ
. It means that for every image of an affine transformation there is a unique pre-image, i.e. there is the inverse transformation. ÿ
Theorem 3. For an affine transformation the image of a straight line is a straight line.
Proof. Let a line 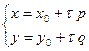 where
where 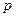 and
and 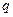 are non-simultaneously equal to zero coordinates of directing vector of the line and an affine transformation
are non-simultaneously equal to zero coordinates of directing vector of the line and an affine transformation  be given. Then the image of the line will be the set of points of the plane with coordinates
be given. Then the image of the line will be the set of points of the plane with coordinates
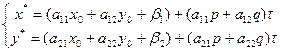 since
since 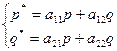 .
.
Observe that if  then we have a line.
then we have a line.
Assume the contrary: let 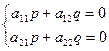 . Since the transformation is affine,
. Since the transformation is affine,  and consequently
and consequently 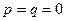 is a unique solution of this system of equations, contradicting our hypothesis. ÿ
is a unique solution of this system of equations, contradicting our hypothesis. ÿ
Theorem 4. For an affine transformation the image of parallel lines are parallel lines, and the point of intersecting the lines-pre-images transfers to the point of intersecting their images.
Proof. Suppose that a pair of parallel lines was transferred by an affine transformation to intersecting or coinciding lines. Consider one of points that are common for the images of lines. Since every affine transformation is bijective, the pre-image of common point is unique and must belong simultaneously to each of the lines-pre-images. However there are no such points since the lines-pre-images are parallel. Consequently, the images of parallel lines are also parallel. If the lines-pre-images are intersected then since every affine transformation is bijective, the image of their point of intersecting can be only the point of intersecting of the images of these lines. ÿ
Theorem 5. For an affine transformation dividing a segment in a given ratio is preserved.
Proof. Let 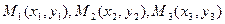 be points lying on some line, and
be points lying on some line, and 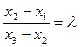 and
and 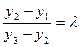 where
where 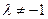 . Let
. Let 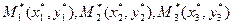 be their images at an affine transformation
be their images at an affine transformation  . It needs to show that
. It needs to show that 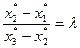 and
and 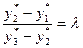 .
.
Let  be given in the form:
be given in the form:  . Then
. Then
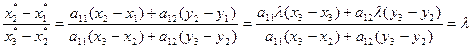 .
.
It is showed analogously that 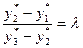 . Observe that from the obtained equalities follow equality of ratio of lengths of images and ratio of lengths of pre-images of segments lying on one line:
. Observe that from the obtained equalities follow equality of ratio of lengths of images and ratio of lengths of pre-images of segments lying on one line: 
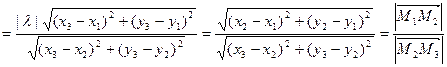 . ÿ
. ÿ
Observe also that Theorem 5 implies that at an affine transformation a segment of a line transfers to a segment.
Theorem 6. For an affine transformation the ratio of lengths of images of two segments lying on parallel lines is equal to the ratio of lengths of their pre-images.
Proof. Let 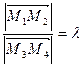 . Draw a line
. Draw a line 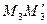 that is parallel to
that is parallel to 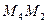 .
.
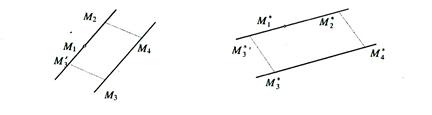
Since at an affine transformation images of parallel lines are parallel, by Theorem 4 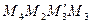 and
and 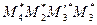 are parallelograms. Consequently,
are parallelograms. Consequently, 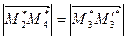 . At last by Theorem 5 we obtain:
. At last by Theorem 5 we obtain: 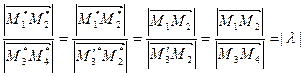 . ÿ
. ÿ
To clarify geometric sense of module and sign of the determinant of a matrix of some affine transformation we give another definition of orientation of pair of non-collinear vectors on plane, transferring consideration of this plane to the space. Let 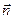 be its some fixed normal vector directed to the direction of an observer. A pair of non-collinear vectors
be its some fixed normal vector directed to the direction of an observer. A pair of non-collinear vectors  and
and 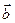 is right (left) oriented if there is
is right (left) oriented if there is 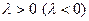 such that
such that 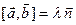 .
.
Theorem 7. 1) At an affine transformation the ratio of the area of the image of parallelogram to the area of the parallelogram itself is equal to the absolute value of 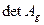 .
.
2) At an affine transformation the orientation of the images of a pair of vectors coincides with the orientation of the pre-images if 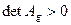 and is replaced on opposite if
and is replaced on opposite if 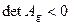 .
.
Proof. Consider some basis formed by vectors 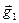 and
and 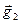 of which the images at an affine transformation
of which the images at an affine transformation  are
are 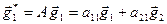 and
and  respectively. The coefficients
respectively. The coefficients 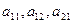 and
and  are elements of the matrix of linear operator
are elements of the matrix of linear operator  , i.e.
, i.e. 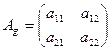 . By properties of vector product the area of parallelogram constructed on basis vectors
. By properties of vector product the area of parallelogram constructed on basis vectors 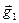 and
and 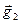
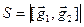 , and the area of parallelogram constructed on the images of basis vectors
, and the area of parallelogram constructed on the images of basis vectors  . We have:
. We have:
 Then
Then 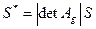 , and the orientation of the pair of vectors
, and the orientation of the pair of vectors 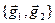 is not changed at
is not changed at 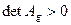 and is replaced on opposite at
and is replaced on opposite at 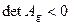 . ÿ
. ÿ
Theorem 8. At an affine transformation every Cartesian system of coordinates transfers to a Cartesian system of coordinates, and the coordinates of the image of every point of plane in new system of coordinates will coincide with the coordinates of the pre-image in the original system.
Proof.
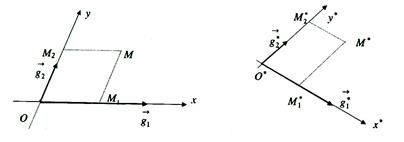
At an affine transformation a basis transfers to a basis. Indeed, let the formulas of a transformation be  . Then the images of old basis vectors are the vectors
. Then the images of old basis vectors are the vectors 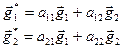 . Since
. Since 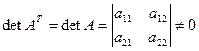 , the vectors
, the vectors  and
and 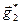 are linearly independent, and consequently we can form a basis. Let in new system the coordinates of
are linearly independent, and consequently we can form a basis. Let in new system the coordinates of 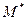 be
be 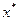 and
and 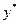 . Then by Theorem 5 the following holds:
. Then by Theorem 5 the following holds:
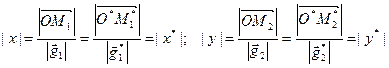 . ÿ
. ÿ
Theorem 9. For every curve of the second order that is not an empty set its type cannot be changed at an affine transformation.
Proof. By Theorem 4 and 6 a parallelogram with its interior transfers to a parallelogram, and consequently, a restricted curve transfers to a restricted one. This implies that ellipses and points can transfer only to ellipses and points. On other hand, a point cannot transfer to an ellipse and conversely since every affine transformation is bijective.
Only hyperbolas and parallel lines among curves of the second order have non-connected branches, i.e. there is a line non-intersecting a curve of the second order such that the branches of this curve are located on different sides from the line. This property is obviously preserved at an affine transformation. Parallel lines cannot transfer to branches of a hyperbola by Theorem 4.
Only parabola among non-straight curves of the second order is a unrestricted connected curve. Consequently, a parabola at an affine transformation can transfer only to a parabola.
If a curve of the second order is a point, a line or a pair of parallel or intersecting lines then by Theorems 3 and 4 follows that their type cannot be changed. ÿ
Дата добавления: 2015-08-27; просмотров: 156 | Нарушение авторских прав
| <== предыдущая страница | | | следующая страница ==> |
| Глоссарий | | | Orthogonal transformations of plane |