Black temperatures

Laws of thermal radiation allow to use as a characteristic of not blackbody so-called black temperature as well as emissivity.

Black temperature is such a conditional value blackbody must have so that its radiation is equal to that of real body. Every body can be characterized by a range of black temperatures depending on kind of radiation.

When comparing total integral radiation fluxes emitted by body having temperature Т and blackbody black temperature is called radiational (Т _r). As follows from definition

or according to Stefan-Boltzmann law

If black temperature is determined on comparison of given body color with that of blackbody it is called color temperature (Т _c). In this case monochromatic radiation for some two wave-length (colors) is usually considered.

According to definition following expression is considered

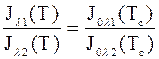. (а)

Using e _l1 and e _l2 in Wien’s law we get

. (b)

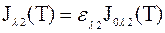. (c)

Substituting expressions (b) and (c) into equation (a) we get

. (d)

Substituting expressions for Plank’s law instead of radiation intensity we get

.

After transformations we get

or .

Taking the logarithm from this expression we obtain

;

;

.

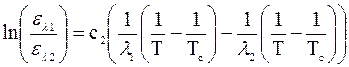So finally we get

.

If intensity of given body monochromatic radiation is compared with that of blackbody then black temperature is called brightness temperature (Т _b). As follows from definition

.

Substituting and using Wien’s law we get relationship between Т and Т _b.

Brightness of monochromatic radiation is

,

where .

Then we get

,

where from .

Taking the logarithm we get

or

.

Дата добавления: 2015-08-17